IMO Shortlist 2010 problem C5
Kvaliteta:
Avg: 0,0Težina:
Avg: 8,0 players participated in a tennis tournament. Any two players have played exactly one game, and there was no tie game. We call a company of four players
players participated in a tennis tournament. Any two players have played exactly one game, and there was no tie game. We call a company of four players 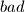 if one player was defeated by the other three players, and each of these three players won a game and lost another game among themselves. Suppose that there is no bad company in this tournament. Let
if one player was defeated by the other three players, and each of these three players won a game and lost another game among themselves. Suppose that there is no bad company in this tournament. Let 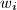 and
and 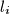 be respectively the number of wins and losses of the
be respectively the number of wins and losses of the 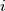 -th player. Prove that
-th player. Prove that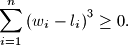
Proposed by Sung Yun Kim, South Korea
Izvor: Međunarodna matematička olimpijada, shortlist 2010
 Školjka
Školjka