IMO Shortlist 2002 problem G7
Kvaliteta:
Avg: 0,0Težina:
Avg: 9,0 The incircle 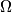 of the acute-angled triangle
of the acute-angled triangle 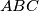 is tangent to its side
is tangent to its side 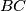 at a point
at a point 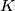 . Let
. Let 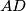 be an altitude of triangle
be an altitude of triangle 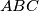 , and let
, and let 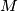 be the midpoint of the segment
be the midpoint of the segment 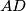 . If
. If 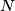 is the common point of the circle
is the common point of the circle 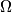 and the line
and the line 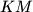 (distinct from
(distinct from 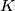 ), then prove that the incircle
), then prove that the incircle 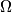 and the circumcircle of triangle
and the circumcircle of triangle 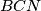 are tangent to each other at the point
are tangent to each other at the point 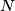 .
.
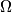 of the acute-angled triangle
of the acute-angled triangle 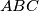 is tangent to its side
is tangent to its side 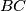 at a point
at a point 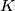 . Let
. Let 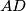 be an altitude of triangle
be an altitude of triangle 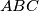 , and let
, and let 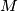 be the midpoint of the segment
be the midpoint of the segment 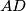 . If
. If 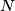 is the common point of the circle
is the common point of the circle 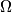 and the line
and the line 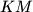 (distinct from
(distinct from 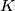 ), then prove that the incircle
), then prove that the incircle 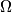 and the circumcircle of triangle
and the circumcircle of triangle 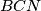 are tangent to each other at the point
are tangent to each other at the point 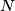 .
. Izvor: Međunarodna matematička olimpijada, shortlist 2002
 Školjka
Školjka