Školjka

There are 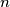$n$ circles drawn on a piece of paper of paper in such a way that any two circles intersect in two points, and no three circles pass through the same point. Turbo the snail slides along the circels in the following fashion. Initially he moves on one of the circles in clockwise direction. Tube always keeps sliding along the current circle until he reaches an intersection with another circle. Then he continues his journey on this new circle and also changes the direction of moving, i.e. from clockwise to anticlockwise or \emph{vice versa}. Suppose that Turbo's path entirely covers all circles. Prove that 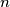$n$ must be odd. \begin{flushright}\emph{(India)}\end{flushright}