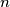
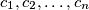
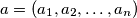
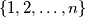
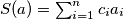
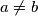
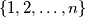
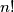
Let be an odd integer greater than 1 and let be integers. For each permutation of , define . Prove that there exist permutations of such that is a divisor of .
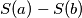
%V0
Let $n$ be an odd integer greater than 1 and let $c_1, c_2, \ldots, c_n$ be integers. For each permutation $a = (a_1, a_2, \ldots, a_n)$ of $\{1,2,\ldots,n\}$, define $S(a) = \sum_{i=1}^n c_i a_i$. Prove that there exist permutations $a \neq b$ of $\{1,2,\ldots,n\}$ such that $n!$ is a divisor of $S(a)-S(b)$.
Izvor: Međunarodna matematička olimpijada, shortlist 2001
Školjka 
be an odd integer greater than 1 and let 
be integers. For each permutation 
of 
, define 
. Prove that there exist permutations 
of 
is a divisor of 
.